The information on this page is ✔ fact-checked.
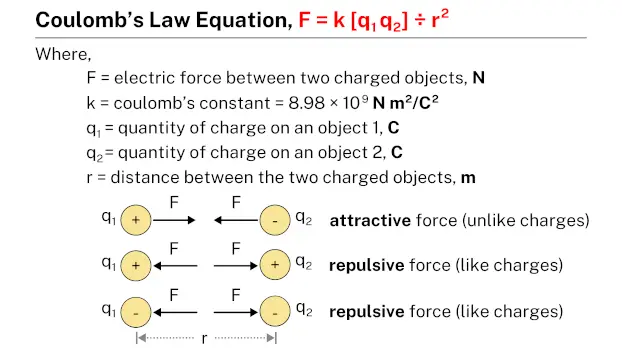
Coulomb’s law describes the electrostatic force between two charged particles. According to this law, the force between two point charges is directly proportional to the product of the magnitudes of the charges and inversely proportional to the square of the distance between them. This force acts along the line connecting the charges and can be attractive if the charges are of opposite signs or repulsive if the charges are of the same sign. For instance, two electrons repel each other due to their like negative charges, while an electron and a proton attract each other because of their opposite charges.
Coulomb’s law is mathematically expressed as:
$$F = k_{e}\frac{\left | q_{1}q_{2} \right |}{r^{2}}$$where F is the magnitude of the force between the charges, q1 and q2 are the amounts of the charges, r is the distance between the centers of the two charges, and ke is Coulomb’s constant, approximately 8.9875 × 109 N m2/C2.
Practice problems
Problem #1
Calculate the electric force between two charged balls, ball 1 and ball 2, which have charges of 12 µC and 16 µC, respectively. The balls are separated by a distance of 1 m. Use the value of Coulomb’s constant, k = 8.98 × 109 N m2/C2.
Solution
Given data:
- Electric force acting between two charged balls, F = ?
- Quantity of charge on ball 1, q1 = 12 µC = 12 × 10-6 C
- Quantity of charge on ball 2, q2 = 16 µC = 16 × 10-6 C
- Distance between the two charged balls, r = 1 m
- Coulomb’s constant, k = 8.98 × 109 N m2/C2
Applying the formula:
- F = k [q1 q2] ÷ r2
- F = [(8.98 × 109) × (12 × 10-6) × (16 × 10-6)] ÷ (1)2
- F = [8.98 × 12 × 16 × 10-3] ÷ 1
- F = 1724.16 × 10-3
- F = 1.72 N
Therefore, the electric force acting between two charged balls is 1.72 N.
Problem #2
Two metal spheres with charges of 25 µC and 6 µC are separated by a distance of 1.1 m. Determine the magnitude of the electric force between these charged spheres, using the value of Coulomb’s constant, k = 8.98 × 109 N m2/C2.
Solution
Given data:
- Quantity of charge on sphere 1, q1 = 25 µC = 25 × 10-6 C
- Quantity of charge on sphere 2, q2 = 6 µC = 6 × 10-6 C
- Distance between the two charged spheres, r = 1.1 m
- Electric force acting between two charged spheres, F = ?
- Coulomb’s constant, k = 8.98 × 109 N m2/C2
Applying the formula:
- F = k [q1 q2] ÷ r2
- F = [(8.98 × 109) × (25 × 10-6) × (6 × 10-6)] ÷ (1.1)2
- F = [8.98 × 25 × 6 × 10-3] ÷ 1.21
- F = 1113.22 × 10-3
- F = 1.11 N
Therefore, the electric force acting between two charged spheres is 1.11 N.
Problem #3
Two charged objects with charges of q1 = 3 µC and q2 = 9 µC are separated by a distance of 2 m. If the value of Coulomb’s constant is k = 8.98 × 109 N m2/C2, calculate the electric force between these two charged objects.
Solution
Given data:
- Quantity of charge on an object 1, q1 = 3 µC = 3 × 10-6 C
- Quantity of charge on an object 2, q2 = 9 µC = 9 × 10-6 C
- Distance between the two charged objects, r = 2 m
- Coulomb’s constant, k = 8.98 × 109 N m2/C2
- Electric force acting between two charged objects, F = ?
Applying the formula:
- F = k [q1 q2] ÷ r2
- F = [(8.98 × 109) × (3 × 10-6) × (9 × 10-6)] ÷ (2)2
- F = [8.98 × 3 × 9 × 10-3] ÷ 4
- F = 60.615 × 10-3
- F = 6.06 × 10-2 N
Therefore, the electric force acting between two charged objects is 6.06 × 10-2 N.
Problem #4
Calculate the electric force between two charged balloons separated by a distance of 1.4 m. The balloons have charges of q1 = 14 µC and q2 = 20 µC. Use the value of Coulomb’s constant, k = 8.98 × 109 N m2/C2.
Solution
Given data:
- Electric force acting between two charged balloons, F = ?
- Distance between the two charged balloons, r = 1.4 m
- Quantity of charge on balloon 1, q1 = 14 µC = 14 × 10-6 C
- Quantity of charge on balloon 2, q2 = 20 µC = 20 × 10-6 C
- Coulomb’s constant, k = 8.98 × 109 N m2/C2
Applying the formula:
- F = k [q1 q2] ÷ r2
- F = [(8.98 × 109) × (14 × 10-6) × (20 × 10-6)] ÷ (1.4)2
- F = [8.98 × 14 × 20 × 10-3] ÷ 1.96
- F = 1282.85 × 10-3
- F = 1.28 N
Therefore, the electric force acting between two charged balloons is 1.28 N.
📝 Your feedback matters. Visit our contact page.
More topics
- Law of conservation of energy
- Newton’s law of universal gravitation
- Newton’s law of cooling
- Coulomb’s law
- Snell’s law
- Ohm’s law
- Hooke’s law
External links
- https://www.physicsclassroom.com/class/estatics/Lesson-3/Coulomb-s-Law
- https://study.com/academy/lesson/magnitude-direction-of-the-electric-force-on-a-point-charge.html
- https://www.omnicalculator.com/physics/coulombs-law
- https://www.studysmarter.us/explanations/physics/fields-in-physics/coulombs-law/
Deep
Learnool.com was founded by Deep Rana, who is a mechanical engineer by profession and a blogger by passion. He has a good conceptual knowledge on different educational topics and he provides the same on this website. He loves to learn something new everyday and believes that the best utilization of free time is developing a new skill.