The information on this page is ✔ fact-checked.
Newton’s law of universal gravitation is a fundamental principle of physics formulated by Sir Isaac Newton. It states that every particle of matter in the universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. This law explains not only the attraction between objects on Earth but also the forces that govern the motions of celestial bodies. For example, it describes how the Earth orbits the Sun and how the Moon orbits the Earth. Newton’s law of universal gravitation was groundbreaking as it provided a unified description of gravity, applicable both on Earth and in the heavens.
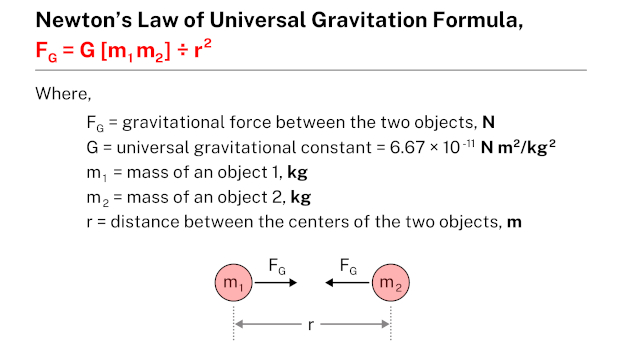
Newton’s law of universal gravitation is mathematically expressed as:
$$F = G\frac{m_{1}m_{2}}{r^{2}}$$where F represents the gravitational force between two objects, G is the gravitational constant, m1 and m2 are the masses of the objects, and r is the distance between their centers. This equation allows us to calculate the gravitational force exerted between objects based on their masses and the distance separating them.
Practice problems
Problem #1
Calculate the gravitational force between a wooden crate weighing 8 kg and a car weighing 100 kg. The distance between the centers of the wooden crate and the car is 200 m. Take the value of the universal gravitational constant, G, as 6.67 × 10-11 N m2/kg2.
Solution
Given data:
- Gravitational force between a wooden crate and a car, FG = ?
- Mass of a wooden crate, m1 = 8 kg
- Mass of a car, m2 = 100 kg
- Distance between the centers of a wooden crate and a car, r = 200 m
- Universal gravitational constant, G = 6.67 × 10-11 N m2/kg2
Applying the formula:
- FG = G [m1 m2] ÷ r2
- FG = (6.67 × 10-11 × 8 × 100) ÷ (200)2
- FG = (6.67 × 10-11 × 8 × 200) ÷ (4 × 104)
- FG = (2668 × 10-11) ÷ 104
- FG = 26.68 × 10-13 N
Therefore, the gravitational force between a wooden crate and a car is 26.68 × 10-13 N.
Problem #2
Determine the gravitational force between two spheres with masses of 4 kg and 10 kg, separated by a distance of 500 m. Take the value of the universal gravitational constant, G, as 6.67 × 10-11 N m2/kg2.
Solution
Given data:
- Gravitational force between the two spheres, FG = ?
- Mass of the sphere 1, m1 = 4 kg
- Mass of the sphere 2, m2 = 10 kg
- Distance between the two spheres, r = 500 m
- Universal gravitational constant, G = 6.67 × 10-11 N m2/kg2
Applying the formula:
- FG = G [m1 m2] ÷ r2
- FG = (6.67 × 10-11 × 4 × 10) ÷ (500)2
- FG = (6.67 × 10-11 × 4 × 10) ÷ (25 × 104)
- FG = (1.06 × 10-11) ÷ 103
- FG = 1.06 × 10-14 N
Therefore, the gravitational force between the two spheres is 1.06 × 10-14 N.
Problem #3
Two satellites, one with a mass of 5000 kg and the other with a mass of 4000 kg, are floating in space. If the distance between them is 2500 m, calculate the gravitational force acting between them. Take the value of the universal gravitational constant, G, as 6.67 × 10-11 N m2/kg2.
Solution
Given data:
- Mass of a satellite 1, m1 = 5000 kg
- Mass of a satellite 2, m2 = 4000 kg
- Distance between the centers of the two satellites, r = 2500 m
- Gravitational force between the two satellites, FG = ?
- Universal gravitational constant, G = 6.67 × 10-11 N m2/kg2
Applying the formula:
- FG = G [m1 m2] ÷ r2
- FG = (6.67 × 10-11 × 5000 × 4000) ÷ (2500)2
- FG = (6.67 × 10-11 × 20 × 106) ÷ (625 × 104)
- FG = (0.2134 × 10-5) ÷ 104
- FG = 0.2134 × 10-9 N
- FG = 21.34 × 10-11 N
Therefore, the gravitational force between the two satellites is 21.34 × 10-11 N.
Problem #4
Determine the gravitational force between two asteroids with masses of m1 = 4 × 1015 kg and m2 = 8 × 1014 kg, located at a distance of 1010 m from each other. Take the value of the universal gravitational constant, G, as 6.67 × 10-11 N m2/kg2.
Solution
Given data:
- Gravitational force between the two asteroids, FG = ?
- Mass of the asteroid 1, m1 = 4 × 1015 kg
- Mass of the asteroid 2, m2 = 8 × 1014 kg
- Distance between the two asteroids, r = 1010 m
- Universal gravitational constant, G = 6.67 × 10-11 N m2/kg2
Applying the formula:
- FG = G [m1 m2] ÷ r2
- FG = (6.67 × 10-11 × 4 × 1015 × 8 × 1014) ÷ (1010)2
- FG = (213.44 × 1018) ÷ 1020
- FG = 213.44 × 10-2
- FG = 2.13 N
Therefore, the gravitational force between the two asteroids is 2.13 N.
📝 Your feedback matters. Visit our contact page.
More topics
- Law of conservation of energy
- Newton’s law of universal gravitation
- Newton’s law of cooling
- Coulomb’s law
- Snell’s law
- Ohm’s law
- Hooke’s law
External links
- https://www.physicsclassroom.com/class/circles/Lesson-3/Newton-s-Law-of-Universal-Gravitation
- http://www.atmo.arizona.edu/students/courselinks/fall12/atmo170a1s1/lecture_notes/mass_weight_density_pressure/univ_grav.html
- https://study.com/academy/lesson/the-law-of-universal-gravitation-definition-importance-examples.html
- https://www.studysmarter.us/explanations/math/mechanics-maths/newtons-law-of-gravitation/
- https://www.omnicalculator.com/physics/gravitational-force
Deep
Learnool.com was founded by Deep Rana, who is a mechanical engineer by profession and a blogger by passion. He has a good conceptual knowledge on different educational topics and he provides the same on this website. He loves to learn something new everyday and believes that the best utilization of free time is developing a new skill.