Ideal gas law

The information on this page is ✔ fact-checked.

The ideal gas law describes the relationship between pressure (P), volume (V), temperature (T), and the number of gas molecules (n). It states that, for an ideal gas, changes in pressure, volume, temperature, and the number of gas molecules are interrelated. This law can simply be understood as a combination of four other gas laws: Boyle’s law, Charles’s law, Gay-Lussac’s law, and Avogadro’s law.

The ideal gas law offers a straightforward method for calculating the physical properties of a given system and provides a fundamental framework for understanding gas behavior. It is based on the assumption of ideal gas behavior, where gas molecules have negligible volume and no intermolecular forces. This law describes how changes in the variables (pressure, volume, temperature, and number of moles) are understood when one variable in the ideal gas law equation (PV = nRT) is unknown or unspecified.

Formula

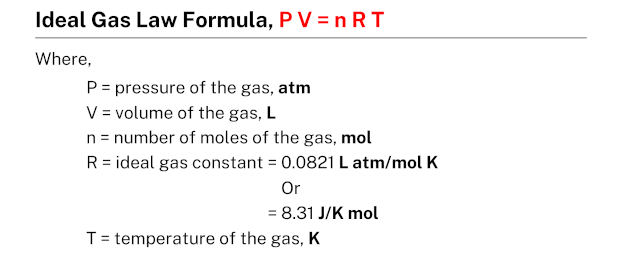
The ideal gas law formula, which is expressed as PV = nRT, provides a relationship between the pressure, volume, temperature, and the number of moles of a given quantity of gas. In this formula, P represents pressure, V represents volume, n represents the number of moles, T represents temperature, and R represents the gas constant. This formula is widely used in physics and chemistry to calculate the properties of gases under different conditions.

Practice problems

Problem #1

Find the number of moles of hydrogen gas in a cylinder with a volume of 4 L, pressure of 2 atm, and temperature of 30 ℃. Use the ideal gas constant value, R = 0.0821 L atm/mol K.

Solution

Given data:

• Number of moles of the gas, n = ?
• Volume of the gas, V = 4 L
• Pressure of the gas, P = 2 atm
• Temperature of the gas, T = 30 ℃ = 303 K
• Ideal gas constant, R = 0.0821 L atm/mol K

Applying the formula:

• PV = nRT
• 2 × 4 = n × 0.0821 × 303
• 8 = n × 24.8763
• n = 0.32 mol

Therefore, the number of moles of the gas is 0.32 mol.

Problem #2

A rubber tire has 0.45 moles of air at a pressure of 12 atm and a temperature of 25 ℃. Calculate the volume of air in the tire. Use the ideal gas constant value, R = 0.0821 L atm/mol K.

Solution

Given data:

• Number of moles of the air, n = 0.45 mol
• Pressure of the air, P = 12 atm
• Temperature of the air, T = 25 ℃ = 298 K
• Volume of the air, V = ?
• Ideal gas constant, R = 0.0821 L atm/mol K

Applying the formula:

• PV = nRT
• 12 × V = 0.45 × 0.0821 × 298
• 12 × V = 11.0096
• V = 1.08 L

Therefore, the volume of the air present in a rubber tyre is 1.08 L.

Problem #3

A cylinder contains 0.3 moles of argon gas and has a volume of 2 L. If the pressure of the gas in the cylinder is 4 atm, what is the temperature of the gas? Use the value of the ideal gas constant, R = 0.0821 L atm/mol K.

Solution

Given data:

• Number of moles of the gas, n = 0.3 mol
• Volume of the gas, V = 2 L
• Pressure of the gas, P = 4 atm
• Temperature of the gas, T = ?
• Ideal gas constant, R = 0.0821 L atm/mol K

Applying the formula:

• PV = nRT
• 4 × 2 = 0.3 × 0.0821 × T
• 8 = 0.0246 × T
• T = 325.20 K

Therefore, the temperature of the gas is 325.20 K.

Problem #4

A container is filled with 6 L of gas containing 1.5 moles of helium gas at a temperature of 20 ℃. Calculate the pressure of the gas in the container. Take the value of the ideal gas constant, R = 0.0821 L atm/mol K.

Solution

Given data:

• Volume of the gas, V = 6 L
• Number of moles of the gas, n = 1.5 mol
• Temperature of the gas, T = 20 ℃ = 293 K
• Pressure of the gas, P = ?
• Ideal gas constant, R = 0.0821 L atm/mol K

Applying the formula:

• PV = nRT
• P × 6 = 1.5 × 0.0821 × 293
• P × 6 = 36.0829
• P = 6.01 atm

Therefore, the pressure of the gas in the container is 6.01 atm.

📝 Your feedback matters. Visit our contact page.

Related

• Gas laws

More topics

• Boyle’s law
• Charles’s law
• Gay-Lussac’s law
• Avogadro’s law
• Ideal gas law
• Dalton’s law
• Henry’s law
• Combined gas law
• Graham’s law

External links

• Ideal gas law – Wikipedia
• What is the ideal gas law? (article) – Khan Academy
• Ideal gas law | Definition, Formula, & Facts – Britannica
• The Ideal Gas Law – Chemistry LibreTexts
• Equation of State – NASA (.gov)
• Ideal Gas Law – HyperPhysics
• What Is the Ideal Gas Law? – WIRED
• Ideal Gas Behavior – StatPearls – National Institutes of Health (.gov)
• The Ideal Gas Law | Physics – Lumen Learning
• The Ideal Gas Law | Equation & Constant – ChemTalk
• Ideal gases and the ideal gas law: pV = nRT – Chemguide
• Empirical Math Model: Ideal Gas Law – Department of Energy (.gov)
• Ideal Gas Law – an overview – ScienceDirect
• PV = nRT: The Ideal Gas Law Fifteen Examples – ChemTeam
• Ideal Gas Law – Texas Gateway
• PV=nRT – Westfield State University
• Gas Laws – Florida State University
• Ideal Gas Law – CK-12
• How to use the Ideal Gas Law | Chemistry – Study.com
• Ideal Gas Law Chemistry Tutorial – AUS-e-TUTE
• An Explanation of the Ideal Gas Law – ThoughtCo