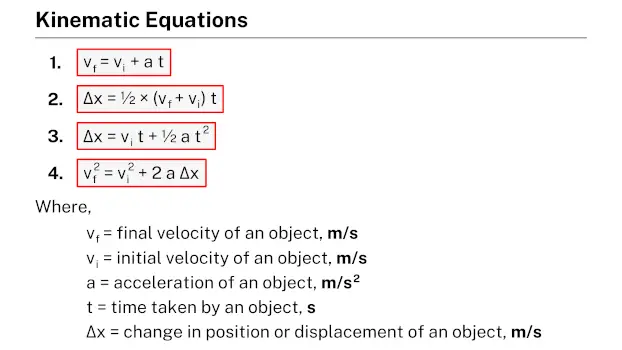
Kinematic equations

Updated on by

The information on this page is ✔ fact-checked.

Kinematic equations
Kinematic equations | Image: Learnool

Kinematic equations describe the motion of objects under constant acceleration. They are fundamental in classical mechanics and are used to predict future motion based on initial conditions. For instance, they can be used to calculate the distance a car travels over a specific time interval if it starts from rest and accelerates at a steady rate. There are four primary kinematic equations that relate displacement (s), initial velocity (u), final velocity (v), acceleration (a), and time (t). Each kinematic equation relates several known variables to calculate the unknown variable, enabling us to determine missing information about an object’s motion based on the given data.

This equation relates the final velocity to the initial velocity, acceleration, and time:

$$v = u + at$$

This equation gives the displacement as a function of initial velocity, acceleration, and time:

$$s = ut + \frac{1}{2}at^{2}$$

This equation relates the final velocity to the initial velocity, acceleration, and displacement:

$$v^{2} = u^{2} + 2as$$

This equation expresses displacement in terms of the average velocity and time:

$$s = \frac{1}{2}\left ( u + v \right )t$$

Tip💡
🡆 Use the first equation when displacement (s) is the missing variable.
🡆 Use the second equation when final velocity (v) is the missing variable.
🡆 Use the third equation when time (t) is the missing variable.
🡆 Use the fourth equation when acceleration (a) is the missing variable.

Practice problems

Problem #1

Calculate the acceleration of a bicycle that goes from 0 m/s to 75 m/s in 15 s.

Solution

Given data:

  • Acceleration of a bicycle, a = ?
  • Initial velocity of a bicycle, vi = 0 m/s
  • Final velocity of a bicycle, vf = 75 m/s
  • Time taken by a bicycle, t = 15 s

Applying the formula, when “Δx” is missing:

  • vf = vi + a t
  • 75 = 0 + (a × 15)
  • 75 = a × 15
  • a = 75/15
  • a = 5 m/s2

Therefore, the acceleration of a bicycle is 5 m/s2.

Problem #2

An athlete participating in the Olympic race accelerates from 4 m/s to 12 m/s in 6 s. Calculate the displacement of the athlete.

Solution

Given data:

  • Initial velocity of an athlete, vi = 4 m/s
  • Final velocity of an athlete, vf = 12 m/s
  • Time taken by an athlete, t = 6 s
  • Displacement of an athlete, Δx = ?

Applying the formula, when “a” is missing:

  • Δx = ½ × (vi + vf) t
  • Δx = ½ × [(4 +12 ) × 6]
  • Δx = 16 × 3
  • Δx = 48 m

Therefore, the displacement of an athlete is 48 m.

Problem #3

A car starts from rest and accelerates at a rate of 15 m/s2 for 4 s. Calculate the displacement of the car during this time interval.

Solution

Given data:

  • Initial velocity of a car, vi = 0 m/s
  • Acceleration of a car, a = 15 m/s2
  • Time taken by a car, t = 4 s
  • Displacement of a car, Δx = ?

Applying the formula, when “vf” is missing:

  • Δx = vi t + ½ a t2
  • Δx = [0 × (4)] + [½ × 15 × (4)2]
  • Δx = ½ × 15 × 16
  • Δx = 120 m

Therefore, the displacement of a car is 120 m.

Problem #4

A boy is riding a bike on the highway with a velocity of 20 m/s. To stop the bike at the gas station, he applies the brakes, causing the bike to decelerate at 8 m/s2. Calculate the displacement of the bike.

Solution

Given data:

  • Initial velocity of a bike, vi = 20 m/s
  • Final velocity of a bike, vf = 0 m/s
  • Deceleration of a bike, a = -8 m/s2
  • Displacement of a bike, Δx = ?

Applying the formula, when “t” is missing:

  • vf2 = vi2 + 2 a Δx
  • (0)2 = (20)2 + [2 × (-8) × Δx]
  • 16 × Δx = (20)2
  • 16 × Δx = 400
  • Δx = 400/16
  • Δx = 25 m

Therefore, the displacement of a bike is 25 m.

More topics

External links

Deep

Learnool.com was founded by Deep Rana, who is a mechanical engineer by profession and a blogger by passion. He has a good conceptual knowledge on different educational topics and he provides the same on this website. He loves to learn something new everyday and believes that the best utilization of free time is developing a new skill.

Leave a Comment