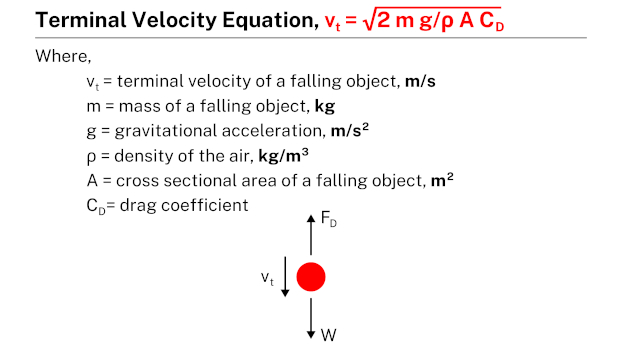
Terminal velocity equation

by

The information on this page is ✔ fact-checked.

Terminal velocity equation
Terminal velocity equation

The terminal velocity equation provides a means to calculate the maximum velocity reached by a falling object when the upward force of air resistance equals the downward force of gravity. It can be expressed as vt = √2 m g/ρ A CD, where vt represents the terminal velocity, m is the mass of the object, g is the gravitational acceleration, ρ is the density of the medium through which the object is falling, A is the cross-sectional area of the object, and CD is the drag coefficient.

Practice problems

Problem #1

What is the terminal velocity of a 0.5 kg parachute bag thrown from a plane? Given the density of the air as 1.21 kg/m3, the cross-sectional area of the parachute bag as 0.10 m2, and the drag coefficient as 0.12. Take the value of gravitational acceleration as g = 9.81 m/s2.

Solution

Given data:

  • Terminal velocity of a parachute bag, vt = ?
  • Mass of a parachute bag, m = 0.5 kg
  • Density of the air, ρ = 1.21 kg/m3
  • Cross sectional area of a parachute bag, A = 0.10 m2
  • Drag coefficient, CD = 0.12
  • Gravitational acceleration, g = 9.81 m/s2

Applying the formula:

  • vt = √2 m g/ρ A CD
  • vt = √(2 × 0.5 × 9.81)/(1.21 × 0.10 × 0.12)
  • vt = √(9.81)/(0.0145)
  • vt = √676.5517
  • vt = 26.01 m/s

Therefore, the terminal velocity of a parachute bag is 26.01 m/s.

Problem #2

When a skydiver weighing 55 kg jumps from a helicopter, he reaches the terminal velocity after traveling a certain distance. Calculate the terminal velocity of the skydiver, considering the density of the air as 1.25 kg/m3, the cross-sectional area of the skydiver as 0.20 m2, and the drag coefficient as 0.70.

Solution

Given data:

  • Mass of a skydiver, m = 55 kg
  • Terminal velocity of a skydiver, vt = ?
  • Density of the air, ρ = 1.25 kg/m3
  • Cross sectional area of a skydiver, A = 0.20 m2
  • Drag coefficient, CD = 0.70
  • Assuming gravitational acceleration, g = 9.81 m/s2

Applying the formula:

  • vt = √2 m g/ρ A CD
  • vt = √(2 × 55 × 9.81)/(1.25 × 0.20 × 0.70)
  • vt = √(1079.1)/(0.175)
  • vt = √6166.2857
  • vt = 78.52 m/s

Therefore, the terminal velocity of a skydiver is 78.52 m/s.

Problem #3

A leaf with a mass of 0.05 kg is floating in the air and descending from a certain height, eventually reaching its terminal velocity “vt.” If the density of the air is 1.22 kg/m3, the cross-sectional area of the leaf is 0.05 m2, and the drag coefficient is 0.08, determine the value of the leaf’s terminal velocity.

Solution

Given data:

  • Mass of a leaf, m = 0.05 kg
  • Density of the air, ρ = 1.22 kg/m3
  • Cross sectional area of a leaf, A = 0.05 m2
  • Drag coefficient, CD = 0.08
  • Terminal velocity of a leaf, vt = ?
  • Assuming gravitational acceleration, g = 9.81 m/s2

Applying the formula:

  • vt = √2 m g/ρ A CD
  • vt = √(2 × 0.05 × 9.81)/(1.22 × 0.05 × 0.08)
  • vt = √(0.981)/(0.0048)
  • vt = √204.375
  • vt = 14.29 m/s

Therefore, the terminal velocity of a leaf is 14.29 m/s.

Problem #4

Calculate the terminal velocity of a 0.012 kg feather floating in the air. Given the density of the air as 1.20 kg/m3, the cross-sectional area of the feather as 0.02 m2, and the drag coefficient as 0.05. Take the value of gravitational acceleration as g = 9.81 m/s2.

Solution

Given data:

  • Terminal velocity of a feather, vt = ?
  • Mass of a feather, m = 0.012 kg
  • Density of the air, ρ = 1.20 kg/m3
  • Cross sectional area of a feather, A = 0.02 m2
  • Drag coefficient, CD = 0.05
  • Gravitational acceleration, g = 9.81 m/s2

Applying the formula:

  • vt = √2 m g/ρ A CD
  • vt = √(2 × 0.012 × 9.81)/(1.20 × 0.02 × 0.05)
  • vt = √(0.2354)/(0.0012)
  • vt = √196.1666
  • vt = 14 m/s

Therefore, the terminal velocity of a feather is 14 m/s.

Related

External links

Leave a Comment